Research topics

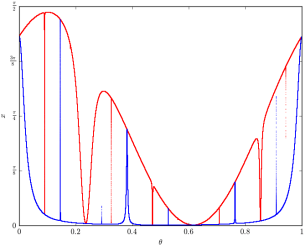
Quasiperiodically forced systems

Quasiperiodic forcing is the simplest type of aperiodic driving that can occur in dynamical systems. In the modelling of real-world phenomena, it comes up whenever the process in question is influenced by two or more periodic external factors with incommensurate frequencies. Examples are multi-frequency forced oscillators or electric circuits and Schrödinger operators with quasiperiodic potential. One phenomenon which has attracted particular interest in qpf systems is the wide-spread occurrence of so-called strange non-chaotic attractors (SNA). These objects combine a complicated structure with non-chaotic dynamics, a combination which is quite rare in dynamical systems otherwise. Another interesting aspect of qpf 1D-maps is that they can be considered either as 1D non-autonomous systems or as autonomous 2D-maps with a special skew product structure. In both situations, they can serve as simple models whose study allows to obtain further insights about the general case.

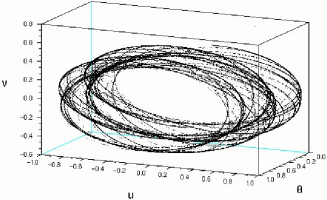Non-autonomous bifurcation theory

One of the primary goals of non-autonomous bifurcation theory is the description of non-autonomous counterparts of the classical bifurcation pattern of dynamical systems. The external forcing often leads to qualitative changes of the bifurcation scenario. For example, saddle-node bifurcations may become non-smooth (thus involving strange non-chaotic attractors) and the usual Hopf bifurcation pattern gives way to the two-step scenario for the non-autonomous Hopf bifurcation proposed by Ludwig Arnold.

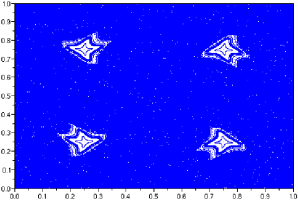Dynamics of surface homeomorphisms

Rotation theory is one of the main tools to understand and classify the dynamical behaviour on surfaces. Originated by Henri Poincare in the context of orientation-preserving circle homeomorphisms and flows on the two-torus, it has further been developed for twist maps of the annulus and homeomorphisms of the torus. However, many fundamental questions still remain open, in particular for the case of torus homeomorphisms.